Math 105--Calculus I: Project 2, Spring 1998

Dealing with small leaks

by Gavin LaRose (glarose@umich.edu), Nebraska Wesleyan University, January 1998

DVI file of project (needs the figure, to be called "98S_p2_f1.eps")
PostScript version of project

The letter from Hocklead Tarmin...

Hocklead-Tarmin, Inc.

27 February 1998

Independent Mathematical Contractors, Inc.
Suite 2, Strawmarket Business Plaza
Lonlinc, SK 04685

Dear IMC:

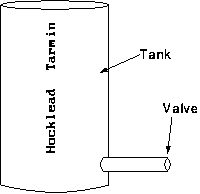
As a primary contractor in defense and the aerospace industry, Hocklead-Tarmin maintains several industrial sites in and around Verden, Docolora. In the course of expanding one of these, we find it expedient to add a number of free standing vertical cylindrical fuel tanks, as shown in figure 1. These are filled and discharged through the valve at the base of tank. As the tanks are large (20' high with radii of 8'), there is a clear incentive to be sure that should they develop a leak the fuel release would be adequately contained, and we are therefore building a wall around each tank that will catch the fuel in this case. Simultaneously, we would like to avoid spending too much on a very large wall if a small one will suffice, and we are therefore contacting you to obtain an estimate for the amount of fuel that might be released.

Figure 1: Fuel tank

The greatest risk of unintended discharge is when the tank is being filled or emptied through the valve (the diameter of which is approximately 9"), as through unintended contact or mechanical failure the valve could fail and allow unimpeded discharge through the pipe to which the valve is connected. In this case, it is known that the rate at which the height of the fuel in the tank will change is proportional to the ratio of the squares of the diameters of the valve and the tank and the square root of the height of the liquid in the tank, with constant of proportionality k=(2g)^1/2 (where g is the acceleration due to gravity).

Owing to our strong accident-containment procedures, any spill in these circumstances should be stopped within 10 minutes of its initiation. We need to know under these conditions how much fuel will need to be contained if the leak starts with a full tank and proceeds for either 5 or 10 minutes.

In order to push the industrial site into production as soon as possible, we need your 3--5 page report by the 16th of March. After careful consideration, we have also contracted with the redoubtable Dr. Gavin LaRose, an errant mathematician in your area, to field any questions you may have in the course of your work. Please note that you must contact him as a team with some indication of your progress by the 4th of March and again by the 11th of March. Failure to meet any of these deadlines will be grounds for partial or complete reduction in your compensation for the project. Dr. LaRose is also able to provide you with example reports should these facilitate your report writing.

We look forward to hearing from you.

Sincerely
O.W.R. Eit III
CEO, Hocklead-Tarmin, Inc.

owre³:glr