Math 224--Differential Equations: Project 2, Spring 1998
Buckles, Beams, and such things...
by Gavin LaRose
(glarose@umich.edu),
Nebraska Wesleyan University, January 1998
©1998 Gavin LaRose (glarose@umich.edu)
permission granted to use and distribute free in an academic setting.
-
- PostScript
version of project
The letter from Rockettech...
Rocket Tech Division
Utoff A.F. Base
1 Piecemeal Drive
Haoma, SK 13681-0050
9 March 1998
Rigorous Mathematical Contractors, Inc.
Suite 3, Strawmarket Business Plaza
Lonlinc, SK 04685
Dear Rimac:
As you know, we have here at the Rocket Tech Division of Utoff
A.F.~Base been involved in the development of the space station
Omfreed, a project for which we have utilized your company's
formidable mathematical expertise in the past. We are currently
considering the structural integrity of the beams that are used to
support different sections of the structure, in an effort to determine
the manner in which they will respond to the rigors of extended use on
the space station.
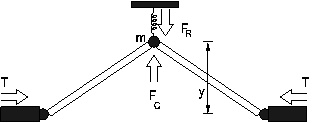The situation which we are considering is shown in figure 1. As seen
there, there is a central mass m (the ball at the center of the
figure) connected to two beams, at the ends of which a force T
pressing inwards is imposed. This results in a force Fc pushing
the mass upwards. The upwards force is opposed by a structural force
in the system, shown in the diagram as Fr. The effect of the force
is to produce a displacement y, which is the deflection of the mass
from an initially horizontal position.
The engineers that have been working on this problem have suggested
that Fr may be modeled as a
nonlinear spring force, Fr =
a(y - y0)2 + b(y-y0)3 +
c(y-y0), where
y0 is the initial position of
the mass (which may be a measure of initial imperfections in the
system) and a, b, and c are constants
characterizing the ``spring.'' The compressional force Fc is given
approximately by Fc = 2 d
y, where d is again a constant---in this case, measuring
the magnitude of the forces T.
Based on this information, we need from you a mathematical model for
the deflection of the mass in figure 1, and based on this a prediction
for what will happen to the mass as the compressional force Fc is
increased. From the work of a resident mathematician who was able to
briefly turn her attention to the project, we expect that you should
be able to write this model in the form
z'' + g z' + z3
+ 2 h z2 + (1 - L)
z - L D = 0
where z(x) is a scaled variable measuring the deflection of
the mass from the initial position y0, g a parameter
measuring the damping internal to the system, h a parameter
measuring the ratio of different components of the restoring force,
D a parameter measuring the initial position of the mass or
system imperfections, and D a parameter measuring the applied
compressional force.
As our manufacturing specifications are at worst exemplary, you should
consider in the greatest depth the case y0=0, and then give some
indication of how the results you obtain change when y0 nonzero. We
would like your results to be as generally stated as possible, but
observe that you may find it expedient in some cases to consider
h = 0.75 and, where appropriate, D = 0.1.
We are under some pressure to forge ahead with the development of
Omfreed, having been beset by delays in other parts of the program.
We therefore need your final 4--10 page report by the 6th of April.
If you should have questions regarding your investigation, please feel
free to contact Dr. Gavin LaRose, a technical expert in many fields,
with whom we have an affiliation for this project through the space
agency. Please note that you should in any event contact him with an
update on your progress on two occasions -- on or before the 13th and
30th of March. Sample report formats are also available from
Dr. LaRose.
We look forward to hearing from you.
Sincerely
Lieutenant General Rick N. Backer
Commander, Rocket Tech Division
rnb:glr
Gavin's DiffEq Project 2, Spring 1998
Last modified: Sun Mar 29 21:20:50 EST 1998
Comments to
glarose@umich.edu