Math 224--Differential Equations: Project 3

defending freedom... or some such

by Gavin LaRose (glarose@umich.edu), Nebraska Wesleyan University, April 1996

Even as it takes money to make money, the fruit of success is further success, and so it appears to be for RiMaC, Inc. After several exceedingly lucrative contracts, further contracts are pouring in. As you eye your departure from RiMaC for greener pastures (all mathematical, of course), you have one final contract to address--the coup de grâce, as it were. And, appropriately enough as you seek to ascend to new heights, it comes from none other than the Utoff A.F. Base, where they are apparently doing some work having to do with the new space station. The letter you received on the subject is attached.

plain TeX file with project -- uses psfig and the eps figure, which should be called "depr3_s96-f.eps" -- or edit the TeX file)
PostScript file of project

The letter...

Rocket Tech Division

7 April 1996

Rigorous Mathematical Contractors (RiMaC), Inc.
Suite 3, Strawmarket Business Plaza
Lonlinc, SK 04685

Dear RiMaC:

As you undoubtedly know, the national space agency is in the process of developing an space station to be built in orbit in the foreseeable future. We have been, on account of our outstanding success in the development of and use of such defensive works as the Otpatri missile system, commissioned to assess the feasibility of designing a system to protect the space station from space asteroids and debris (of which there is an increasing amount in orbit!).

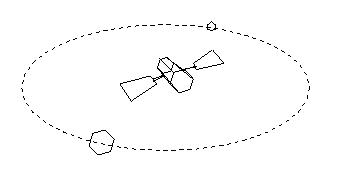
Our current plan is to put two defense units in orbit around the space station Omfreed, positioned as shown in Figure 1, 180 degrees apart. Then, if an asteroid approaches the space station, the closest defense unit will fire a rocket to remove all chance of harm to the station.

Figure 1
Figure 2

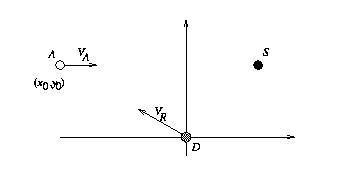
To keep the required resources to a minimum, we are first considering a very simple guidance system for the defense rocket in which the rocket maintains an orientation which always points towards the asteroid. As shown in Figure 2, this is true at the point at which the asteroid is first found to be a problem ($(x_0, y_0)$ in Figure 2), and will be true at all points thereafter.[1]

To keep things specific, we would like to consider the situation shown in Figure 2, where V_A = 5 miles/sec, V_R = 20 miles/sec, and the x and y distances from the asteroid to the defense unit are 15 miles and 5 miles, respectively. Suppose that the space station S is one mile (in the x direction) from the defense unit and in line with the asteroid. In this case, will the defense unit succeed in protecting the space station? Does it matter whether V_R is much larger than V_A?

Owing to the necessity that we be able to review the results of your analysis before submitting them to the national space agency, we must require your finished project be submitted by the 29th of April. If you should have questions regarding your investigation, please feel free to contact Dr. Gavin LaRose, a technical expert in many fields, with whom we have an affiliation for this project through the space agency. Please note that you should in any event contact him with an update on your progress on two occasions -- on or before the 15th and the 22nd of April. We look forward to seeing the results of your work.

Yours sincerely,
Lieutenant General Rick N. Backer
Commander, Rocket Tech Division

[1] Your tremendously useful physical measurements department has suggested that this may have something to do with the slope, dy/dx, of the rocket's path, and that the x and y velocities of the rocket x'(t) and y'(t) will have something to do with it as well.

Back to Differential Equations Projects Page

To Differential Equations Labs Page

To NWU Differential Equations Resource Page

To Gavin's Home Page

last modified on 8 Apr 1996