
First let's think about the slice. We visualize this below:

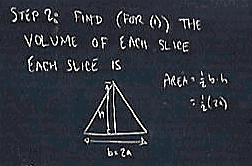
The first thing we need to do to find the volume of our object is to find the surface area of one slice. These slices are equilateral triangles with side length of 2a. To find the area, use the formula, A= (1/2)bh. We know the base so now we need to find the height of the triangle, which we can do by using the pythagorean theorem.
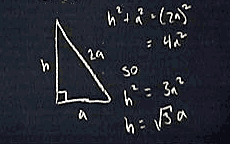
After we find the height of the triangle, we can find the area. Just plug the numbers into the appropriate places! We find the area to be (sqrt(3))(a^2).
Now that we have an equation for the volume of the figure, we need to integrate this equation to find the exact volume of the figure. Because the length of the figure is L, we can integrate the area from 0 to L: