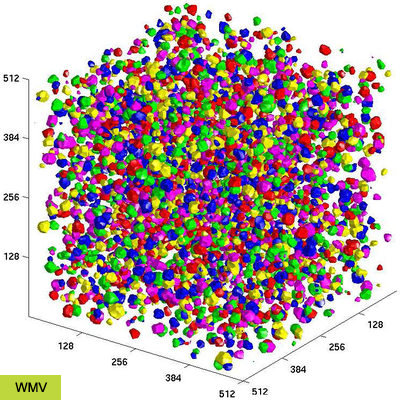
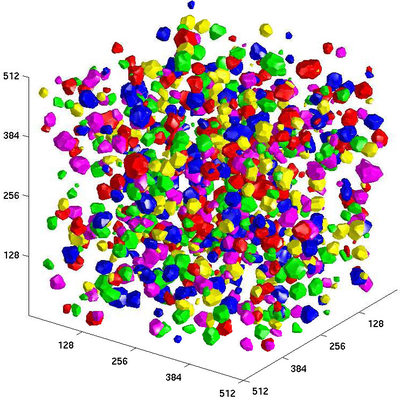
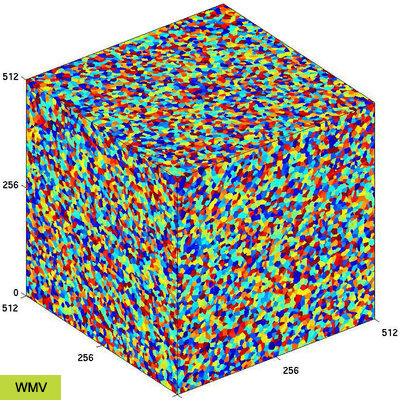
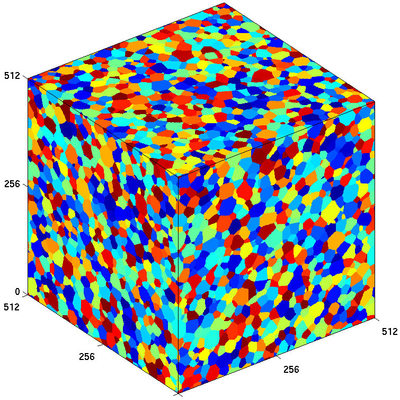
Motion of Grain Boundaries in Polycrystalline Materials

Many common materials, such as most metals and ceramics, are polycrystalline: They are made up of tiny crystallites called grains that are distinguished from their neighbors by their differing crystallographic orientation. When these materials are heated (i.e. annealed) -- for instance during a manufacturing process -- grain growth occurs: The network of grains decreases its energy through a coarsening procedure, which involves the growth of some of the grains at the expense of others. Statistical measures of the grain network, such as the grain size distribution, have important implications for the macroscopic properties of the material, such as its conductivity and brittleness. As such, simulating how the grain network evolves is of great technological interest. We developed new, efficient, and accurate numerical methods for simulating grain growth and related dynamics. This work has been supported by NSF grant DMS-0748333.

Publications and Preprints:

  1. Elsey, M.; Esedoglu, S.; Smereka, P. Diffusion generated motion for grain growth in two and three dimensions. UCLA CAM Report 09-39 (April 2009). Journal of Computational Physics. 228:21 (2009), pp. 8015-8033.

  2. Elsey, M.; Esedoglu, S.; Smereka, P. Large scale simulation of normal grain growth in two and three dimensions. Proceedings of the Royal Society A: Mathematical, Physical, and Engineering Sciences. 467:2126 (2011), pp. 381-401.

  3. Elsey, M.; Esedoglu, S.; Smereka, P. Large scale simulations and parameter study for a simple model of recrystallization. Philosophical Magazine. 91:11 (2011), pp. 1607-1642.

  4. Esedoglu, S. Large-scale simulations of grain boundary motion in polycrystals. SIAM News. 43:8 Oct. 2010.

  5. Elsey, M.; Esedoglu, S.; Smereka, P. Simulations of anisotropic grain growth: effiicent algorithms and misorientation distributions.Accepted for publication in Acta Materialia.

3D Simulation Results:

The individual grains shown below are from a 512x512x512 simulation of grain growth (joint work with Matt Elsey and Peter Smereka), using the distance function based diffusion generated motion algorithm.

The initial condition for the simulation contained 133,110 grains. Below, a large number of the grains are shown at 100 (left image) and 300 (right image) iterations. Not all the grains are shown, since they fill up the entire volume.

The full grain pattern is shown below, viewed from the faces of the computational domain. Periodic boundary conditions were imposed. On the left is the initial condition; on the right, the solution at 300 time steps is shown.