Dimers in Combinatorics and Cluster Algebras
August 3-14, 2020
University of Michigan
"Dimers in Combinatorics and Cluster Algebras" will take
place online in the weeks of August 3-7 and 10-14, 2020.
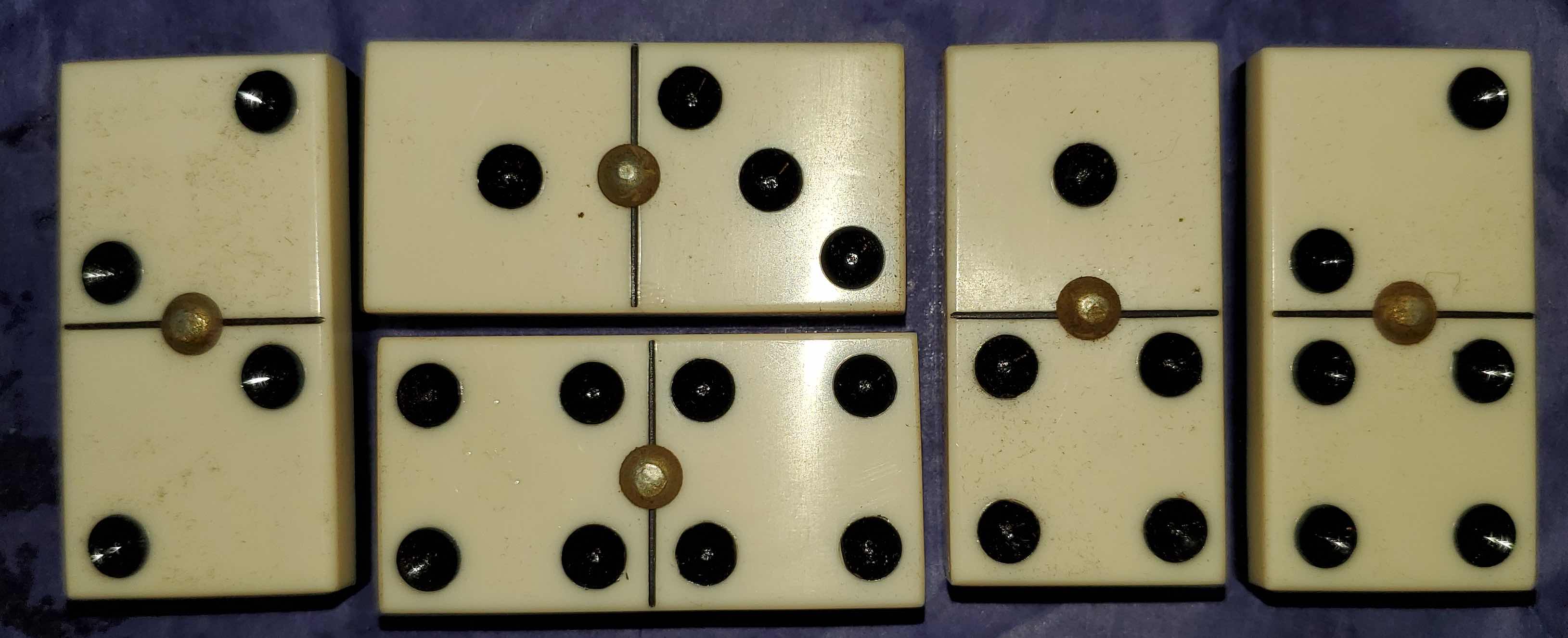
All talks will take place at https://umich.zoom.us/j/91300032965
. The password is the number of ways to tile a 2x5 rectangle with 2x1
dominos, as in the image below. The dominos should be treated as
indistinguishable; in other (less fun) words, we are counting dimer covers of a
2x5 rectangle. The password should be written as a number, not spelled
out with letters. You can also e-mail an organizer for the
password.
We will have informal conversations at 1 PM on conference days.
Our current plan is to do this using Gather. The Gather link is https://gather.town/IFS50ReKfPx3xXnl/DIMERS,
the password is the same as for the Zoom meeting. We strongly
recommend not being on Zoom and Gather at the same time. See this
google document for more information.
Our schedule for each day is:
- 10:00-10:40 AM Expository talk
- 11:00-11:40 AM Research talk
- 12:00-12:40 PM Research talk
- 1:00 PM - ??? Informal discussions
All times and dates are given in Ann Arbor Michigan, which is in the US
Eastern Time Zone and will be observing Daylight Savings Time.
This conference is targeted at people who use dimers in a range of
combinatorial areas, including exact enumerations of dimer covers,
parametrizations of Grassmannians and positroid cells, cluster
algebras, Schur processes and limit shapes, discrete integrable
systems, dimer models in quantum field theory and non-commutative
resolutions of toric singularities; we aim to welcome graduate
students and young researchers begining work in these fields.
Organizers:
Sebastian Franco (City College of New York), Richard Kenyon (Yale), Gregg Musiker (University of Minnesota), David E Speyer (University of Michigan), Lauren Williams (Harvard)
Confirmed Speakers:
| Expository: | | Research: | | | |
| Karin Baur | University of Leeds | Raf Bocklandt | University of Amsterdam | Greg Muller | University of Oklahoma |
| Phillippe Di Francesco | University of
Illinois at Urbana-Champaign | Mihai
Ciucu | Indiana University | Matthew Pressland | University of Leeds |
| Thomas Lam | University of Michigan | Sergey Fomin | University of Michigan | Jim Propp | UMass Lowell |
| Alex Postnikov | MIT | Pavel Galashin | UCLA | Marianna Russkikh | MIT |
| | Helen Jenne | University of Oregon | Sibylle Schroll | Univerisity of Leicester |
| | Ray Karpman | Otterbein | Jeanne Scott | Universidad de los Andes |
| | Alastair King | University of Bath | Harold Williams | University of Southern California |
| | Tri Lai | University of Nebraska | Benjamin Young | University of Oregon |
Schedule:
| Monday, August 3: | | |
| 10:00-10:40 (Expository) | Alex
Postnikov | Dimers and
Grassmannian (slides)
(video) |
| 11:00-11:40 | Ray Karpman | The purity conjecture for
symmetric plabic graphs (slides) (video) |
| 12:00-12:40 | Pavel Galashin | Symmetries of stochastic
colored vertex models (slides)
(video) |
| Tuesday, August 4: | | |
| 10:00-10:40 (Expository) | Alex Postnikov | Dimers and Grassmannian (slides) (video) |
| 11:00-11:40 | Sergey Fomin | Expressive curves (slides) (video) |
| 12:00-12:40 | Harold Williams | Kasteleyn operators from mirror
symmetry (slides) (video) |
| Wednesday, August 5: | | |
| 10:00-10:40 (Expository) | Phillippe Di
Francesco | Cluster Algebra, Dimers and Beyond (slides) (video) |
| 11:00-11:40 | Jeanne Scott | Perturbing Isoradial
Triangulations (slides) (video) |
| 12:00-12:40 | Jim Propp | Reciprocity, Quasirandomness, and Resonance (slides) (video) |
| Thursday, August 6: | | |
| 10:00-10:40 (Expository) | Phillippe Di
Francesco | Cluster Algebra, Dimers and Beyond (slides) (video) |
| 11:00-11:40 | Marianna Russkikh | Dimers and embeddings (slides) (video) |
| 12:00-12:40 | Greg Muller | Spaces of quasiperiodic sequences (slides) (video) |
| Monday, August 10: | | |
| 10:00-10:40 (Expository) | Thomas
Lam | Dimers and webs (slides) (video) |
| 11:00-11:40 | Helen Jenne | Combinatorics of the double-dimer
model (slides) (video) |
| 12:00-12:40 | Benjamin Young | The combinatorial PT-DT correspondence (video)
|
| Tuesday, August 11: | | |
| 10:00-10:40 (Expository) | Thomas
Lam | Dimers and webs (slides) (video) |
| 11:00-11:40 | Mihai Ciucu | The effect of microscopic gap
displacements on the correlation of gaps in dimer systems on the
hexagonal lattice (slides) (video)
|
| 12:00-12:40 | Tri Lai | Tiling Shuffling Phenomenon (slides) (video) |
| Wednesday, August 12: | | |
| 10:00-10:40 (Expository) | Karin Baur | Dimer models with boundary, Grassmannian cluster categories and friezes (slides) (video)
|
| 11:00-11:40 | Alastair King | Categorification of perfect matchings (slides) (video) |
| 12:00-12:40 | Sibylle Schroll | Grassmannian categories of infinite rank (slides) (video) |
| Thursday, August 13: | | |
| 10:00-10:40 (Expository) | Karin Baur | Dimer models with boundary, Grassmannian cluster categories and friezes (slides) (video)
|
| 11:00-11:40 | Matthew Pressland | From dimers in the disk to
cluster categories (slides) (video) |
| 12:00-12:40 | Raf Bocklandt | Curved Dimers (slides) (video) |
Abstracts
Karin Baur
To dimer models with boundary we associate dimer algebras (frozen Jacobian algebras). In certain cases, these algebras correspond to cluster-tilting objects
in the cluster category associated to the Grassmannian introduced by Jensen, King and Su. We give various sources for such dimer models (surface triangulations, tilings, strand diagrams) and explain how they provide friezes.
We introduce orbifold diagrams which as quotients by a rotational symmetry. We use these to give a construction of skew group categories over the Grassmannian
cluster category. (sildes 1) (slides 2) (video 1)
(video 2)
Raf Bocklandt
To a dimer model with weights on the vertices of the quiver, we assign a curved gentle A_infty algebra.
The representation theory of this curved algebra can be used to model relative Fukaya categories
of closed surfaces. We explain the details of this construction and its relation to specular duality for dimer models. (slides) (video)
Mihai Ciucu
In earlier work we showed that the correlation of gaps in dimer
systems on the hexagonal lattice is governed, in the fine mesh limit,
by Coulomb's law for 2D electrostatics. We also proved that the
scaling limit of the average tile orientation is the electric field
produced by a system of charges corresponding to the gaps. In this
talk we show that the effect of microscopic gap displacements on the
correlation of gaps is also controlled by the electric field of the
corresponding system of charges. (slides) (video)
Phillippe Di Francesco
We use the T-system/Octahedron equation to describe the connections
between cluster algebra, matrix representations, flat connections,
non-intersecting paths on networks and dimer/domino tilings.
We will first describe the toy model of the A1 T-system, and then generalize it.
In a second part we show how to use the cluster algebra formulation to
access asymptotic properties of dimers such as the arctic circle phenomenon.
Finally we will describe an alternative, seemingly unrelated method to
compute arctic curves, the so-called Tangent Method, which has the advantage
of applying to a wider range of systems, including
interacting/osculating paths. (slides) (video 1) (video 2)
Sergey Fomin
We call a real plane algebraic curve C expressive if its defining
polynomial has the smallest number of critical points allowed by the
topology of the set of real points of C. We give a necessary and
sufficient criterion for expressivity (subject to a mild technical
condition), describe several constructions that produce expressive
curves, and relate their study to the combinatorics of plabic graphs,
their quivers and links. This is joint work with E. Shustin. (slides) (video)
Pavel Galashin
The stochastic colored six-vertex model is a simple statistical
mechanical model defined in terms of pipe dreams. We discover a new
hidden symmetry of the model called flip-invariance, which generalizes
recent shift-invariance results of Borodin-Gorin-Wheeler. Our proof
relies on an equivalence between the stochastic colored six-vertex
model and the Yang-Baxter basis of the Hecke algebra. We conclude by
discussing the relationship of the model with Kazhdan-Lusztig
polynomials and positroid varieties in the Grassmannian.
(slides) (video)
.
Helen Jenne
We will discuss a new result about the double-dimer model: the
partition function for tripartite double-dimer configurations of a
planar bipartite graph satisfies a recurrence related to Dodgson
condensation (also called the Desnanot-Jacobi identity). A similar
identity for the number of dimer configurations of a planar bipartite
graph was established nearly 20 years ago by Kuo. Our work was
motivated in part by its potential for applications. In the following
talk, Ben Young will discuss an application to a problem in
Pandharipande-Thomas and Donaldson-Thomas theory. (slides) (video)
Ray Karpman
A collection of k-element subsets of {1,2,…,m} is weakly separated if
for any two members I and J of the collection, when the integers
1,2,…,m are arranged in a circle, there is a chord separating I∖J from
J∖I. Oh, Postnikov and Speyer proved a correspondence between maximal
weakly separated collections and Postnikov's plabic graphs, which give
coordinate charts on the Grassmannian of k-planes in m-space. In this
talk, we show that maximal "symmetric" weakly separated collections
give coordinate charts on the Lagrangian Grassmannian of maximal
isotropic subspaces with respect to a symplectic form. We note that
Danilov, Karzanov and Koshevoy extended one of our main results in a
preprint from July 2020. (slides) (video)
Alastair King
I will explain several consequences of thinking about perfect
matchings as modules for a dimer algebra. The context will be, as far
as possible, for the dimer model on a disc associated to a general
Postnikov diagram. The main goal is to relate the Marsh-Scott
partition function to the cluster character formula. (slides) (video)
Tri Lai
"The modifications in size and orientation of the holes in a punctured region would lead to unpredictable changes in the tiling generating function. However, in some particular cases, the tiling generating function is changed by only a simple multiplicative factor. More interestingly, in these cases, the generating functions maybe not simple products. In other words, there are many `sibling' regions, whose tiling generating functions are not given by closed-form formulas, but the ratio of their tiling generating functions is an elegant product formula. We call this the
`tiling shuffling phenomenon.' In this talk, I will present various
examples of the phenomenon. (slides) (video)
Thomas Lam
A bipartite graph embedded into a disk gives rise to a point in a Grassmannian Gr(k,n), obtained by counting dimer configurations with various boundary conditions. I will discuss the interplay between the coordinate ring of the Grassmannian and the combinatorics of dimers.
One construction of the coordinate ring of the Grassmannian uses a
planar graphical model, known as "webs". I will explain a duality
between the combinatorics of dimers and of webs, based on joint work
with Chris Fraser and Ian Le. (slides) (video 1) (video 2)
Greg Muller
A "quasiperiodic space" is a vector space of sequences which are
periodic up to a constant factor. The moduli of such vector spaces are
1-dimensional extensions of Grassmannians, and there are analogous
positroid stratifications of the former. I will demonstrate that these "quasiperiodic positroid varieties" have a Y-type cluster structure that is mirror dual to the X-type cluster structure on (the Plucker cone over) the corresponding positroid variety. This structure is defined by extending a version of Postnikov's boundary measurement map to the quasiperiodic case.
Time permitting, I will discuss an alternative construction of this
boundary measurement map, which uses the twist to construct a linear
recurrence whose solutions are the space in question. This provides a
generalization of MGOST's connection between linear recurrences,
friezes, and the Gale transform. A motivating goal of this project is
to understand the tropical points of these quasiperiodic positroid
varieties, as they parametrize the canonical basis of theta functions
on (the Plucker cone over) the corresponding positroid variety." (slides) (video)
Alex Postnikov
We will give an introduction to the positive Grassmannian
and discuss its links with dimers, plabic graphs, positroids,
membranes, and related combinatorial structures. (slides) (video 1) (video 2)
Matthew Pressland
A consistent dimer model on the disk determines a cluster algebra structure on the coordinate ring of a positroid variety in the Grassmannian. I will explain how the dimer model can also be used to give a categorification of this cluster algebra, as a result of certain Calabi-Yau symmetries in the dimer algebra. (slides) (video)
Jim Propp
In this suite of mini-talks I'll advertise three unrelated topics that
deserve more attention. Why do formulas for the number of perfect
matchings in certain families of graphs display symmetries suggestive
of an Ehrhart-type reciprocity? If most random domino tilings of an
Aztec diamond manifest a circular arctic region, why is it so hard to
give a deterministic recipe for one that does without “cheating”? When
one introduces dynamics on the set of lozenge tilings of a regular
hexagon of side n, why do so many orbits have size divisible by
3n-1? (slides) (video)
Marianna Russkikh
We establish a correspondence between dimer models on a bipartite graph and circle patterns with the combinatorics of that graph. This correspondence is the key for studying Miquel dynamics, a discrete integrable system on circle patterns. We describe how to construct a 't-embedding' (or a circle pattern) of a dimer planar graph using its Kasteleyn weights, and develop a relevant theory of discrete holomorphic functions on t-embeddings; this theory unifies harmonic functions on T-graphs and s-holomorphic functions coming from the Ising model.
Based on: joint works with D. Chelkak, R. Kenyon, W. Lam, B. Laslier and S. Ramassamy. (slides) (video)
Sibylle Schroll
We give a categorification of the coordinate rings of Grassmannians of infinite rank in terms of graded maximal Cohen-Macauley modules over a hypersurface singularity. This gives an infinite rank analogue of the Grassmannian cluster categories introduced by Jensen, King, and Su. We show that there is a structure preserving bijection between the generically free rank one modules in a Grassmannian category of infinite rank and the Plücker coordinates in a Grassmannian cluster algebra of infinite rank. For this we will introduce a new combinatorial tool, which we call staircase paths, which determine the dimensions of the extension spaces of two generically free rank one modules. This is joint work with Jenny August, Man-Wai Cheung, Eleonore Faber and Sira Gratz. (slides) (video)
Jeanne Scott
In joint work with François David, we consider three discrete
operators — the Beltrami-Laplace operator, the David-Eynard Kähler
operator, and the conformal Laplacian— each defined over a general
Delaunay triangulation. When the triangulation is isoradial all three
operators coincide with the critical Laplacian but otherwise they are
distinct. Using R. Kenyon's formula for the Green's function of the critical Laplacian, we formally
compute a perturbative expansion of the log-determinant of each
operator over a Delaunay triangulation obtained by smoothly deforming
the embedding of a given isoradial triangulation.
For the Beltrami-Laplace and Kähler operators, the second order term
in this expansion has an asymptotic form which admits a continuum
limit whose value is independent of the initial isoradial
triangulation. (Note: The same is true in the case of the conformal
Laplacian however some regulation on the density of pairs of concyclic
triangles in the initial isoradial triangulation must be imposed.)
This continuum limit can be interpreted in light of the Operator
Product Expansion in conformal field theory; in particular a "central
charge" can be identified. (slides) (video)
Harold Williams
In this talk we explain an interpretation of the Kasteleyn operator of
a doubly-periodic bipartite graph from the perspective of homological
mirror symmetry. Specifically, given a consistent bipartite graph G in
T^2 with a complex-valued edge weighting E we show the following two
constructions are the same. The first is to form the Kasteleyn
operator of (G,E) and pass to its spectral transform, a coherent sheaf
supported on a spectral curve in (C*)^2. The second is to take a
certain Lagrangian surface L in T^* T^2 canonically associated to G,
equip it with a brane structure prescribed by E, and pass to its
homologically mirror coherent sheaf. This lives on a toric
compactification of (C*)^2 determined by the Legendrian link which
lifts the zig-zag paths of G (and to which the noncompact Lagrangian L
is asymptotic). As a corollary, we obtain a complementary geometric
perspective on certain features of algebraic integrable systems
associated to lattice polygons, studied for example by
Goncharov-Kenyon and Fock-Marshakov. This is joint work with David
Treumann and Eric Zaslow. (slides) (video)
Benjamin Young
In this talk we describe a combinatorics problem that arises in algebraic geometry - namely, that the topological vertices in Donaldson-Thomas theory and Pandharipande-Thomas theory are equal. We prove a combinatorial version of this conjecture, by translating to the single- and double- dimer models, respectively, and then applying the double-dimer model condensation techniques developed by Jenne. (video)
Registration:
Please fill out this poll to register for the conference. As will be obvious, this poll was set up before the COVID situation developed, but please still use it. Thanks!
Workshop supported by NSF DMS-1854225.